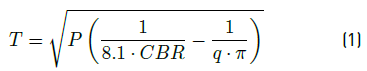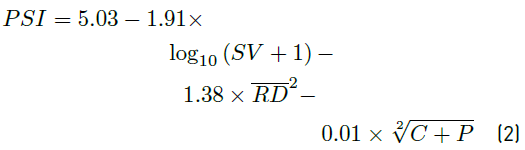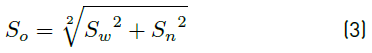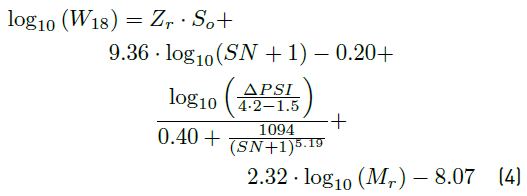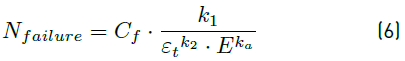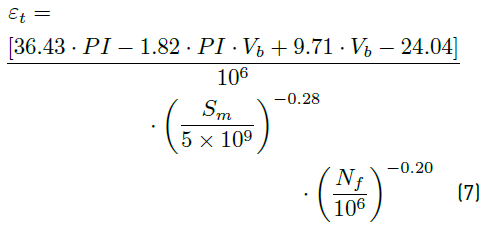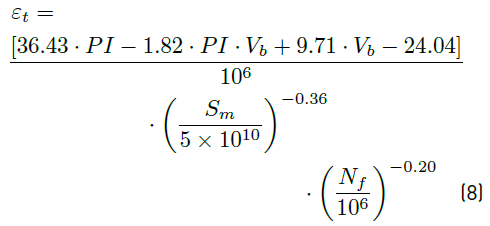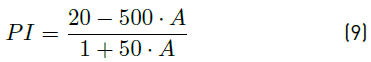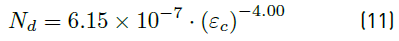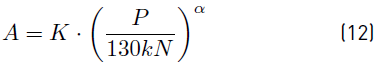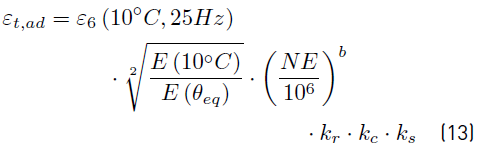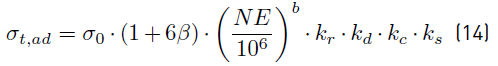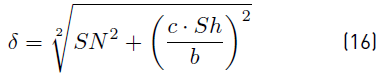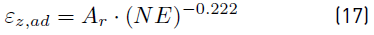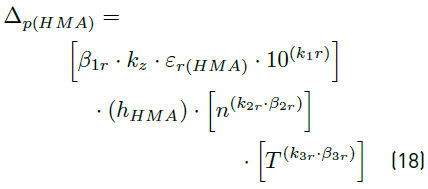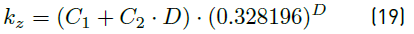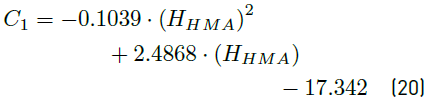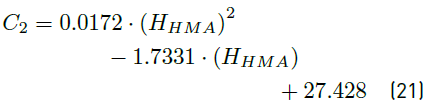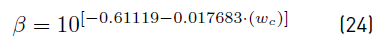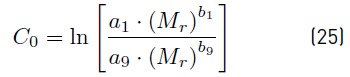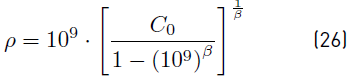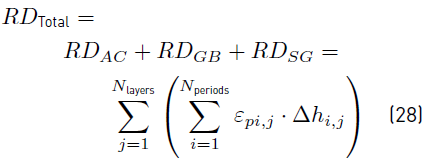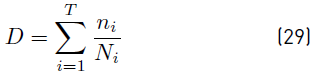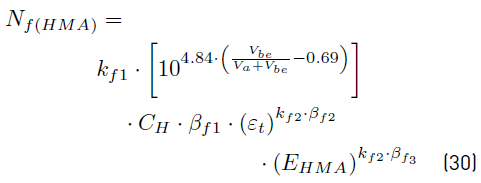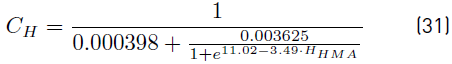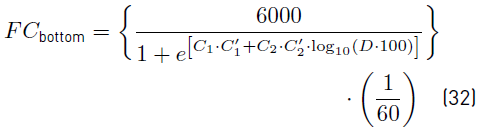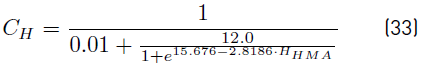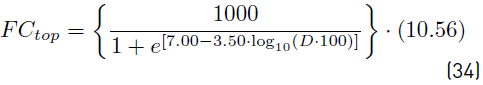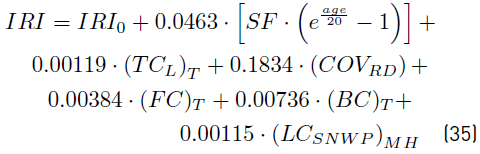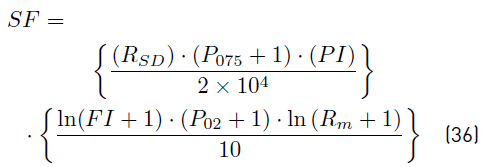1. Introduction
Asphalt pavements are made up of multiple layers: subgrade, unbound subbase and base, hydraulic-binder or asphalt-treated bases, asphalt concrete bases, and wearing courses. All these materials show a complex response to load and environmental changes. Each region or country may follow a particular pavement design guide, but they all must conform to the same basic design principles: considering load repetitions and environmental cycles, and relating the theoretical and the real behavior of materials and structures [1]. Traffic characterization and forecasting, pavement materials and subgrade assessments, environmental effects, and life-cycle economic analysis are challenging in all pavement projects regardless of their longitude, intended purpose (road, port, or airport), and strategic value (local, collector or highway). This article presents an overview of asphalt pavement design for streets and roads, divided into four sections; this Introduction is the first one. The second section introduces the geotechnical framework and the design principles of asphalt pavements in streets and roads. The third section summarizes the evolution of the structural design of asphalt pavements from the empirical approaches to the current mechanistic-empirical methodologies, and the fourth section summarizes the views of the authors about the subject.
2. The geotechnical framework and the design principles of asphalt pavement design
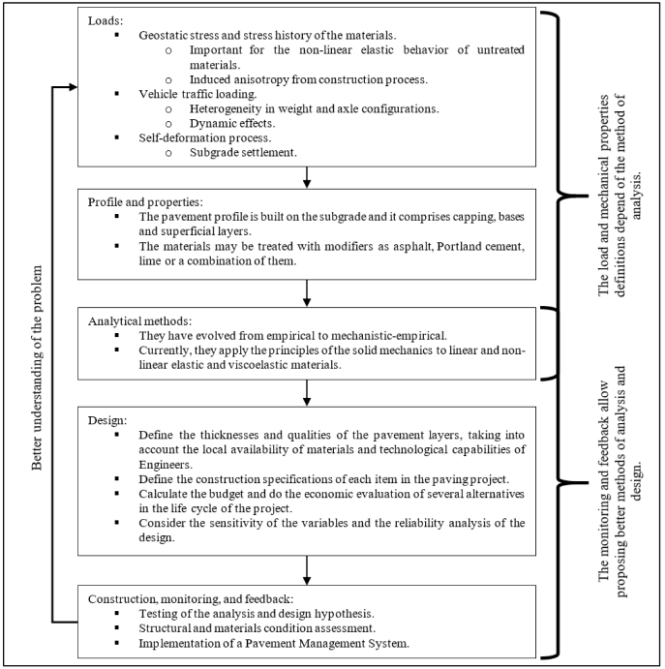
Pavements constitute a geotechnical problem since they are built on the ground and with materials obtained from it: untreated, such as soils and rocks, and processed as hydraulic and bituminous binders. People built pavements over the terrain, on cuts, embankments, even inside tunnels, or at the bottom of human-made ponds. Pavement materials include natural and artificial constituents as soil, rock, lime, Portland cement and bitumen, polymers and geo-synthetics, and a variety of chemical products to enhance their natural characteristics. Even though pavement failure usually does not compromise life or property, pavements are challenging structures and require a systemic approach to include all the activities related to analysis, design, construction, and monitoring. Pavement management systems or modern asset management systems also include the economic aspects of pavement engineering [2].
Figure 1 shows the five constitutive elements of pavement as a geotechnical problem. This article focuses on the “Design” of asphalt pavements for streets and roads, the evolution of the design methods, and the current trends.
Pavement design consists of two broad categories: (a) design of asphalt mixtures and hydraulic-binder-treated materials, and (b) structural design of the pavement components, which is different from the structural design of bridges and buildings since environmental factors greatly influence the pavement structure. Serviceability and the intended use of the pavement are the main topics in designing a pavement structure [3].
Pavement design must consider the expectations of the users since the dynamic interaction between the vehicle and the pavement substantially affects their valuation of the structure. The design of functional pavements must focus on the satisfaction of the users considering five primordial aspects: velocity, smoothness, safety, maintenance, and cost. Therefore, a systematic approach to pavement design must consider the “optimization of the pavement” when all the components (subgrade, structure, wearing course) have the same structural reliability, including their general response to environmental conditions. The design problem has a cost associated with reliability, which also affects the construction, maintenance, operation, and contingency costs in the life cycle of the project [4].
3. Evolution of the asphalt pavement design methods for streets and roads
The structural design of pavements evolved from “art to science;” however, empiricism is indispensable to calibrate the behavior of in-service structures with the responses of the analytical models. The modern asphalt pavements appeared at the end of the nineteenth century, built by combining techniques developed a century before with innovative materials in the upper layers to water-proof the structure and control the dust production. Pavement design methods comprise two main groups: empirical methods and mechanistic-empirical methods, also called analytical or rational methods [5].
3.1 Empirical methods
The empirical methods include those based on the subgrade engineering classification (Bureau of Public Roads), the relative shear resistance of the soil (CBR), and road experiments such as the WASHO Road Test (1952-1955) and the AASHO Road Test (1958-1962). The design method considers the observed behavior of in-service pavements under traffic, environmental, and materials conditions similar to those existing in observed sections [6].
The method based on the California Bearing Ratio test
The method based on the CBR test (ASTM D1833) began with the work in Soil Mechanics in the California State Highway Division. Later on, the United States Army Corps of Engineers (USACE) developed Equation 1 to correlate the CBR, the pavement thickness, and the magnitude of the wheel load based on calibration studies during World War II [7].
Where T is the total thickness of the pavement in inches (1 inch = 25.4 mm), P is the applied wheel load in pounds (1 lb = 4.4482 newtons), CBR is the California Bearing Ratio in percent, and q is the tire pressure in psi (1 psi = 6.8948 kPa).
Figure 2 shows the evaluation of Equation 1 for three levels of wheel load and tire pressure of 224 psi (1682 kPa), which represents the landing gear of a World War II bombardier.
In the CBR method, pavement fails because of permanent deformation due to excessive shear stresses in the subgrade. Therefore, the design principle of the CBR method is to protect the subgrade with a minimum thickness of material with better quality than the on-site soil. The main limitation of the CBR method is that it does not take into account the strength of the upper layers in the pavement structure. After the publication of the AASHO Road Test results, Road Agencies modified the CBR method to characterize the vehicular traffic as repetitions of an 18-kips standard single axle based on several assumptions [8].
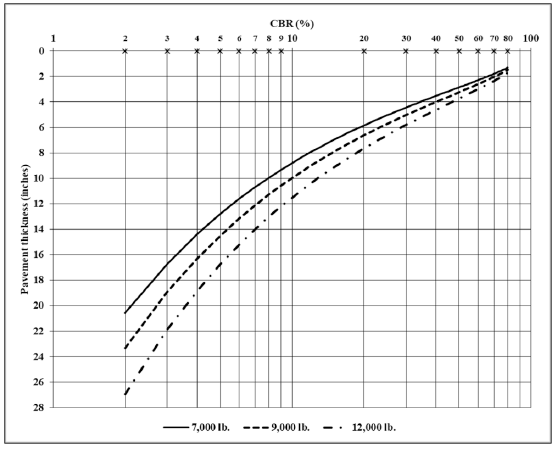
Figure 3 shows the 1979 National Association of Australia State Road Authorities (NAASRA) pavement design method for traffic intensities up to 50 million repetitions of the AASHTO standard axle of 80 kN and CBR values between two and 30 percent. Later on, Austroads updated this chart to design pavements with thin asphalt surfacing [9].
American Association of State Highways and Transportation Officials method
The AASHTO method is based on the statistical analysis of pavement behavior data obtained in the AASHO Road Test (1958-1960), a full-scale facility built in Ottawa, IL. (USA). Although the origin of the method has a robust empirical component, its successive versions incorporated mechanistic analysis to interpret and extrapolate the results from the Road Test on asphalt pavements. In the latest version, the design method incorporated mechanistic concepts into a "semi-empirical" approach that considers two design criteria: serviceability and reliability [10].
Serviceability, or Present Serviceability Index (PSI), was developed in the 1950s from the subjective evaluation of multiple pavement sections with the Present Serviceability Rating and its statistical correlation with measurable characteristics like roughness and distress (cracking, deformation, patching).
Theoretically, PSI varies between zero (a failed pavement) and five (a good pavement); however, the AASHO Road Test data indicates that asphalt pavements may be in the range from 4.2 to 1.5 or less. Equation 2 shows the original relationship between the PSI and several measurable characteristics of asphalt pavements:
Where RD is the average rut depth in inches (1 inch = 25.4 mm) on both wheel tracks, and SV is the slope variance of the average slope of both wheel tracks (x106) measured with the CHLOE profilograph. C is the cracked area with an alligator pattern, and P is the patched area, both in square feet over 1,000 ft² (1 ft² = 0.0929 m²).
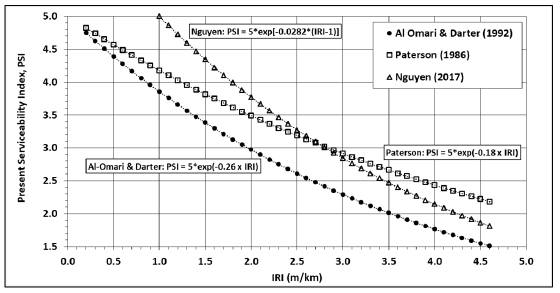
Roughness has a significant effect on PSI, and, at present, it is represented as the effect of the road profile over a standard vehicle, i.e., the International Roughness Index (IRI). For example, Figure 4 shows three correlations between PSI and IRI that substitute the use of Equation 2 .
Reliability considers two sources of uncertainty in pavement design: (a) the prediction of the future traffic on the structure, and (b) the prediction of the serviceability of the pavement in any future moment after supporting the traffic loading. Both uncertainties are standard deviations of the logarithm of the accumulated standard 18-kip single-axle loads. Thus, the overall standard deviation (S o ) is given by Equation 3:
Where S w is the standard deviation of the traffic estimation and S n is the standard deviation of the pavement condition assessment.
The structural design of asphalt pavements with the 1993 AASHTO method applies the pavement behavior algorithm given by Equation 4 [10]:
Where W
18 is the expected number of repetitions of the standard single axle load of 18-kips
on the design lane for the design period of analysis; Z
r
is the accumulated value of the standardized normal distribution for a given reliability
level; S
o
is as previously defined in Equation 3; SN is the “structural number,” an abstract representation of the pavement equal to the summation of the products
of the layer thickness (D
i
, in inches) times a non-dimensional coefficient that represents the layer quality
(a
i
), [
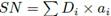 ]; ΔPSI is the estimated loss of serviceability in the service period; and M
r
is the resilient modulus of the pavement materials or subgrade in psi (1 psi = 6.8948
kPa).
]; ΔPSI is the estimated loss of serviceability in the service period; and M
r
is the resilient modulus of the pavement materials or subgrade in psi (1 psi = 6.8948
kPa).
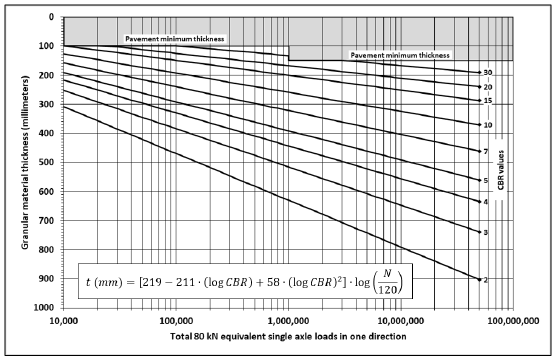
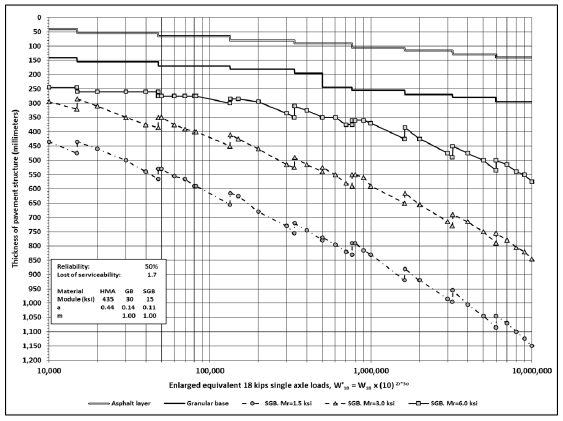
Figure 5 shows a design chart for flexible asphalt pavements with a reliability of 50%. The chart includes three values of resilient moduli of the subgrade: 1,500 psi, 3,000 psi, and 6,000 psi. The properties of the materials are summarized in the same chart. The thicknesses are in millimeters to ease comparison with Figure 3. The increase in layer thickness follows the same tendency for the method based on the CBR test. There are some discontinuities in the granular subbase thickness curves due to the minimum requirements of thicknesses for the asphalt and granular base layers in the AASHTO method.
Engineers still use the 1993 AASHTO method in the design of both new pavements and the rehabilitation of existing ones. However, part of the design practice is based only on the CBR test, and practitioners apply the AASHTO method with several uncertain presumptions and correlations for local or regional conditions.
3.2 Mechanistic-empirical methods
Method of analysis
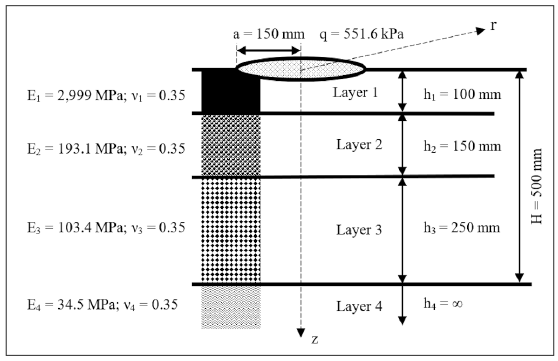
The mechanistic-empirical method became useful with the introduction of the layered elastic theory in 1943 [13]. However, only until the 1962 “First International Conference on the Structural Design of Asphalt Pavements,” the theory gained attention as a valid method of analysis for pavements [14]. The initial application of layered elastic theory considered systems with two or three layers simplified in tables and charts [3]. The two and three-layers solutions focused on measurable structural responses calibrated with the in-service behavior of asphalt pavements as the surficial deflection or the vertical stress. The analysis of road and airfield pavements extensively uses the layered elastic theory (LET). LET models the structure as a semi-infinite continuum, divided into layers of finite thickness over an elastic half-space, as shown in Figure 6.
LET employs boundary pressures to simulate the effect of wheel loads. Early works computed the vertical stresses under parabolic loads with superposition to overcome the inaccuracy of constant contact pressures [15]. The extension of the LET differential equations allows considering vertical, centripetal-horizontal, unidirectional-horizontal, and rotational-horizontal loads [16]. A uniformly distributed rectangular model, with normal and shear components, is a more realistic approach to the tire footprint according to the tensile-strains in the asphalt concrete and compressive-strains in the subgrade [17]. Besides the load representation, there are proven issues about the capacity of LET to represent real stresses and strains in pavements [18].
Alternatives for the layered elastic theory
Method of equivalent thickness: The method of the equivalent thickness (MET) allows the fast computation of responses by transforming a multi-layered media to a single layer with a transformed thickness increased (or decreased) by a factor near to the one-third power of the modular ratio. The method assumes the equality of the rigidity against flexural stress with adjustment factors for better agreement with layered elastic solutions [19].
Probabilistic stress distribution: This method predicts the stress distribution in flexible pavements with the central limit theorem of probability and a coefficient of lateral stress for each material. The linear-elastic solution is a particular case of this approach without assumptions about the transference of boundary energy at the interfaces [20].
Supervised learning algorithms: Artificial neural networks (ANN) and support vector machines (SVM) can estimate the tensile strain in asphalt layers and compressive strain in the subgrade under a standard axle load of 80 kN. Both techniques give coefficients of determination higher than 0.99 in training and testing tests with a synthetic database of N-layer LET solutions [21, 22].
Finite element method (FEM): The layered theory has limitations such as the inability to capture the exact load geometry and boundary conditions. The evaluation of 2D, axisymmetric, and 3D FEM computations indicates that axisymmetric models give a reasonable solution in terms of computation time and capabilities to problems unaffected by boundary or discontinuity conditions [23]. The main advantage of FEM is that it considers elastic non-linear or viscoelastic materials, as well as complex load conditions. The disadvantages are that FEM requires more computational processing time and more information about the behavior of the materials.
The method of analysis is not the pavement design procedure by itself. In pavement design, the challenges are in the mechanical characterization and calibration of the behavior of the materials with real-life conditions.
The basis of the conventional mechanistic-empirical design
The fundamental principles of the structural design of asphalt pavements consider two primary modes of distress:
Permanent deformation: Permanent, plastic, or irrecoverable deformation of the pavement structure appears in the surface as ruts or surface depressions in the trajectory of the wheels. This damage, known as “rutting” [24], is due to two leading causes: (1) the plastic deformation of the unbounded materials due to the change in volumetric phases by secondary compaction under traffic, or by general shear failure in the foundation soil; (2) the plastic deformation of asphalt mixes with excessive amounts of binder or subjected to high temperatures.
Rutting is a compound failure mode; however, there is an empirical model that relates the unrecoverable surface deformation with the elastic response of the subgrade defined by Equation 5:
Where N
d
is the allowable number of load repetitions until the development of a rut of a certain
depth, and
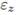 is the vertical compressive strain at the top of the subgrade due to the traffic
loading. The values k
4 and k
5 are obtained with regression analysis from the observation of in-service pavements.
There is a substantial dispersion in the published models because of the differences
in the rut depth defined as failure threshold and the discard of the real contribution
of each layer to the total plastic deformation of the pavement. At present, it is
accepted that the rutting estimation in all the layers of the pavement must be part
of any rigorous mechanistic-empirical design methodology [25].
is the vertical compressive strain at the top of the subgrade due to the traffic
loading. The values k
4 and k
5 are obtained with regression analysis from the observation of in-service pavements.
There is a substantial dispersion in the published models because of the differences
in the rut depth defined as failure threshold and the discard of the real contribution
of each layer to the total plastic deformation of the pavement. At present, it is
accepted that the rutting estimation in all the layers of the pavement must be part
of any rigorous mechanistic-empirical design methodology [25].
Fatigue cracking: Fatigue cracking is a series of interconnecting cracks caused by fatigue failure of the asphalt concrete surface under repeated traffic loading [24]. The properties of asphalt materials change throughout the service life of the pavement with the aging of the bitumen caused by environmental conditions. Materials treated with hydraulic binders (Portland cement, lime, fly ash, and slag) also present changes in their mechanical properties throughout the service life. The traditional fatigue model considers that cracking starts in the bottom of layers due to tensile stresses under traffic loading, i.e., “bottom-up cracking;” however, research indicates that fatigue cracking tends to develop from top to bottom, or “top-down,” and that the opposite is less frequent on in-service pavements [26].
The horizontal tensile strain correlates with the fatigue behavior of the asphalt layers and depends primarily on the layer thickness and the rigidities of the asphalt and base layers. Equation 6 represents the fatigue behavior of asphalt materials:
Where N
failure
is the number of load repetitions to fatigue failure of the asphalt material subjected
to a repetitive horizontal strain (
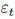 ) at a temperature and load conditions that mobilize a specific Young’s modulus (E). The values k
1, k
2 and k
3, are obtained from laboratory fatigue tests and the C
f
coefficient calibrate the controlled conditions in the laboratory with the real behavior
of in-service pavements.
) at a temperature and load conditions that mobilize a specific Young’s modulus (E). The values k
1, k
2 and k
3, are obtained from laboratory fatigue tests and the C
f
coefficient calibrate the controlled conditions in the laboratory with the real behavior
of in-service pavements.
The general procedure of the mechanistic-empirical design
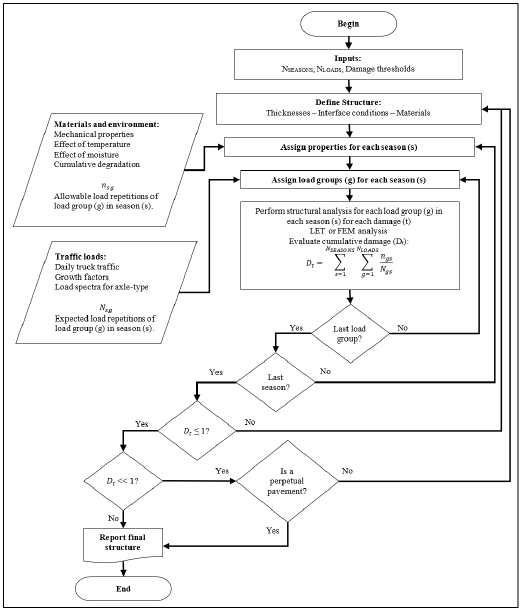
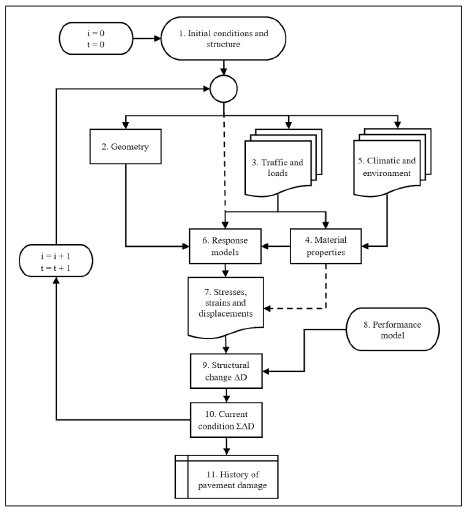
Figure 7 shows a flowchart for a mechanistic-empirical (M-E) pavement design procedure with multiple seasons (climatic sub-periods) and several load groups.
The conventional M-E design considers one season, with weighted or critical climatic values, and a single load group of repetitions of a standard axle. The consideration of multiple climatic seasons and several load groups by type (single, tandem, triple, and quad) and magnitude (load spectra) defined the basis of the incremental design method even before the development of the MEPDG.
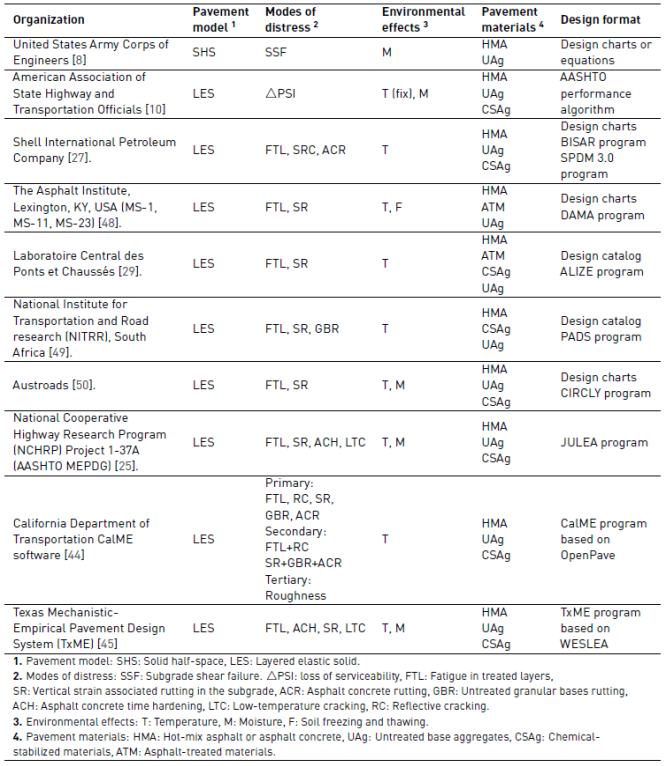
The following paragraphs present some general aspects of two classic M-E methodologies, Shell Oil and the French Design Method, and the basics of the MEPDG for flexible pavements. The influence of the latter methodology is growing in practice despite its demands for extensive activities of calibration and materials characterization.
Shell Oil method: The first edition of the Shell method dates from 1963. Later on, the “Shell Pavement Design Manual (SPDM)” of 1978 and its 1985 addendum replaced it. The method is based on the layered elastic theory and presents the design alternatives in charts and tables. The last version of the Shell method, known as SPDM 3.0, is a software suite published in 1993 without any known updates to date [27].
The SPDM considers a three-layer structure composed of asphalt materials, untreated granular bases, and the subgrade soil. The mechanical properties of each material are Young´s modulus (E) and Poisson´s ratio (v). Repetitions of a 40 kN semi-axle load with dual wheels of 105 mm of radius, center-to-center separation of 315 mm, and contact pressure of 577 kPa represent the traffic loading [27].
The modes of distress are fatigue cracking and permanent deformation of the asphalt layers, and permanent deformation associated with the subgrade elastic response. The expected life of the pavement is the allowable repetitions of the standard axle load based on the tensile or compressive strains in the materials.
Equations 7 to 11 summarize the design criteria of the Shell Oil method [5]:
a. Fatigue of bituminous mixtures based on constant stress tests:
b. Fatigue of bituminous mixtures based on constant strain tests:
In both Equations 7 and 8,
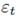 is the tensile strain, PI is the bitumen penetration index, V
b
is the percentage of by bitumen volume in the mix, S
m
is the stiffness modulus of the mix in N/m², and N
f
is the number of repetitions to failure. Equation 9 defines the penetration index of the bitumen (PI):
is the tensile strain, PI is the bitumen penetration index, V
b
is the percentage of by bitumen volume in the mix, S
m
is the stiffness modulus of the mix in N/m², and N
f
is the number of repetitions to failure. Equation 9 defines the penetration index of the bitumen (PI):
Where A is the temperature susceptibility of the bitumen, i.e., the slope of the line plot between the logarithm of penetration (pen = 0.1 mm) and temperature (°C) as defined by Equation 10:
c. Rutting associated with the subgrade response as given by Equation 11:
Where N
d
is the number of load repetitions to failure, defined as a 13 mm rut depth, with
a reliability of 50% reliability, and
 is the vertical compressive strain at the top of the subgrade.
is the vertical compressive strain at the top of the subgrade.
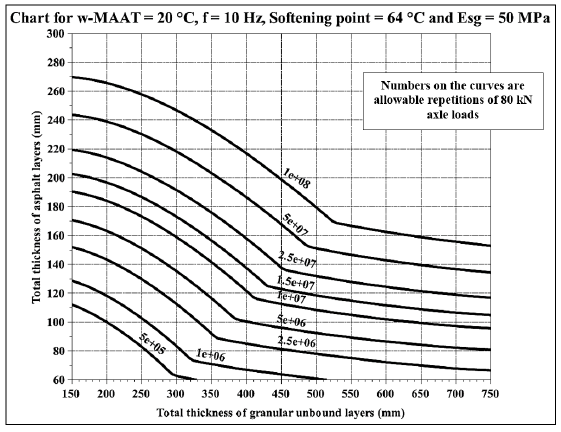
Figure 8 shows a design chart based on the Shell procedure for structures on a subgrade with Young’s modulus of 50 MPa and a weighted mean annual air temperature of 20°C. The asphalt mix has the mechanical properties of a dense-graded mix or S1-F1-100 in Shell codification. The horizontal axis represents the thickness of untreated base layers, and the vertical axis represents the thickness of the asphalt concrete layers. The chart includes nine traffic levels of repetitions of the 80 kN axle load. The rutting controls the structures with small thicknesses of unbound base and asphalt while the fatigue of the asphalt mix controls the thicker ones.
Practicing engineers used the Shell procedure for decades as the archetype of the mechanistic-empirical design method before the development of the MEPDG. The Shell method capabilities increased with the conversion of the analogic charts into the SPDM 3.0 software.
The French Design Guide: After World War II, the French design practice applied the experimental procedures based on the CBR test. The increase in heavy traffic and the use of new materials in road construction required the development of new design methods in the 1970s. As in the Shell Oil method, the French procedure uses (1) charts based on mechanical analysis with the layered elastic program Alizé, and (2) in situ performance criteria based on the compressive strain of the roadbed and deflection of the surface [28].
The current French design method combines an analytical method to compute stresses and strains, and the results of laboratory tests to assess the fatigue resistance of pavement materials. Also, two significant features of the method are: (1) the detailed consideration to the design of embankments or capping layers on the subgrade; and (2) the inclusion of a risk assessment of pavement design considering the random mechanical response of the materials [28]. The different axle loads and configurations are converted to repetitions of a single axle of 130 kN, applied by two wheels with a radius of 125 mm, center to center spacing of 375 mm, and contact pressure of 662 kPa. Equation 12 is used to transform any axle to the standard axle [29]:
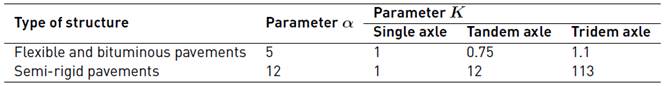
Where A is the “aggressiveness” of any given axle, K is used to take into account the kind of axle, P is the axle weight in kN, and α is a function of the type of material and structure. Table 1 summarizes the values of α and K for French conditions on regular and highly trafficked new flexible and semi-rigid pavements:
Equations 13 to 17 summarize the design criteria of the French method [28] and [29]:
a. Fatigue of bonded or cemented materials: For asphalt pavements, the allowable strain is defined by Equation 13:
Where
 is the allowable tensile strain in the asphalt layer;
is the allowable tensile strain in the asphalt layer;
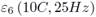 is the tensile strain of the fatigue law at 10°C, 25 Hz, and 106 load repetitions; E (θ
eq
)is the elastic modulus of the asphalt mixture at the equivalent temperature (θ
eq
); NE is the number of load repetitions to failure with a 50% probability, and b is the slope of the bi-logarithmic fatigue law. For pavements with hydraulic-binder-treated
bases or concrete slabs, the allowable stress is defined by Equation 14:
is the tensile strain of the fatigue law at 10°C, 25 Hz, and 106 load repetitions; E (θ
eq
)is the elastic modulus of the asphalt mixture at the equivalent temperature (θ
eq
); NE is the number of load repetitions to failure with a 50% probability, and b is the slope of the bi-logarithmic fatigue law. For pavements with hydraulic-binder-treated
bases or concrete slabs, the allowable stress is defined by Equation 14:
Where σ
t,ad
is the allowable tensile stress at the bottom of tensile-stressed layers; σ0 is the indirect tensile strength of the material; β is the slope of the Wohler’s
curve (σ/ σ0=1+β·logN); NE is the number of load repetitions to failure with a 50% probability; and  for load repetitions between 105 and107. In Equations 13 and 14, the coefficients k
r
, k
c
, k
s
, and k
d
modify the allowable strain or stress considering the reliability, the type of pavement,
the modulus of the subgrade, and the type of materials treated with hydraulic binders,
respectively. The following paragraphs present their definitions.
for load repetitions between 105 and107. In Equations 13 and 14, the coefficients k
r
, k
c
, k
s
, and k
d
modify the allowable strain or stress considering the reliability, the type of pavement,
the modulus of the subgrade, and the type of materials treated with hydraulic binders,
respectively. The following paragraphs present their definitions.
The coefficient k r , defined in Equation 15, adjusts the allowable strain or stress values to the design reliability:
Where Z r is the accumulated value of the standardized normal distribution for a given reliability (or risk) level, b is the slope of the bi-logarithmic fatigue law, and δ is the standard deviation associated with the load repetitions given the dispersion of the fatigue law and the layer thickness as shown in Equation 16:
Where SN is the standard deviation of the logarithm of the number of cycles at failure, Sh is the standard deviation of the layer thickness, c is the coefficient linking the variation in strain (or stress) to the random variation
in thickness
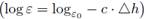 , and b is the slope of the bi-logarithmic fatigue law.
, and b is the slope of the bi-logarithmic fatigue law.
The calibration coefficient K c adjusts the allowable strain or stress to the performance observed on pavements of the same type. The coefficient K s is a reducing factor that takes into account the heterogeneity of the bearing capacity of the roadbed. The coefficient k d takes into account the effect of joints or shrinkage cracks in cement-treated materials or concrete slabs. Table 2 summarizes the suggested values of the coefficients for French conditions.
b. The rutting associated with the untreated layers is evaluated with Equation 17:
Where
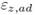 is the allowable compressive strain on the top of untreated or subgrade layers; A
r
is 0.012 in pavements with medium or high traffic and 0.016 in pavements with low
traffic, and NE is the number of load repetitions to failure.
is the allowable compressive strain on the top of untreated or subgrade layers; A
r
is 0.012 in pavements with medium or high traffic and 0.016 in pavements with low
traffic, and NE is the number of load repetitions to failure.
The French pavement design method “is the most comprehensive design method in use in Europe,” [30] and its suitability to any local practice depends on the field calibration and the adoption of construction specifications that evaluate the mechanical performance of the materials. Figure 9 shows the ideal design procedure proposed by the “Advanced Models for Analytical Design of European Pavement Structures - AMADEUS” report. The incremental European approach anticipated the MEPDG by several years; however, it is not implemented to date.
Mechanistic-Empirical Pavement Design Guide (MEPDG): The MEPDG is the main product of the NCHRP 1-37A project for the implementation of mechanistic-empirical pavement analysis and design procedure. A mechanic model computes structural responses that consider material properties, environmental conditions, and loading-characteristics of the vehicles. The structural responses are analyzed with empirical models to evaluate the performance and predict the distress of the pavement. The accuracy of the empirical models to predict damage depends on the quality of the field data obtained to calibrate them [25].
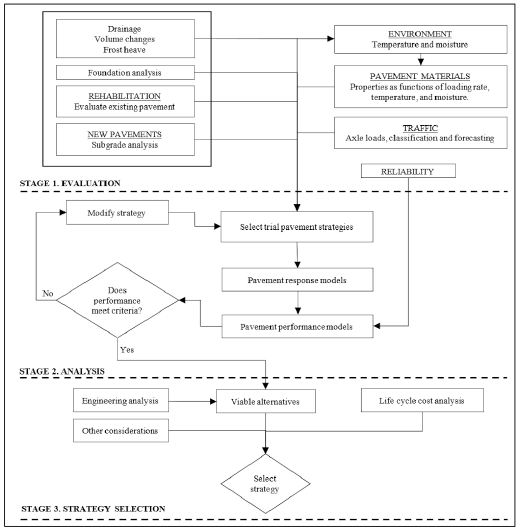
Figure 10 shows the conceptual schematic of the design process of MEPDG divided into three stages: evaluation, analysis, and strategy selection.
The method considers two types of models: one that directly predicts the damage (rutting), and one that predicts the damage from calibrated observations (fatigue). The design process differs from conventional methods because the result is the estimated distress of the structure and not the thicknesses of the layers.
The MEPDG considers three hierarchical levels for the input data: The Level 1 demands high-quality laboratory characterization of the materials, the Level 2 uses correlations between simple parameters and advanced material characterization, and the Level 3 uses local or national values by default. The hierarchical level must coincide with the relevance of the project. The initial calibration of the method corresponds to Level 3, and, in consequence, the road agencies must develop local studies [32].
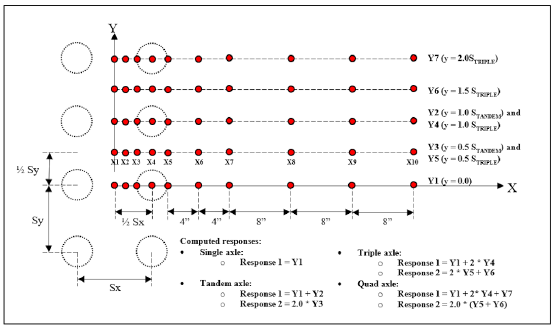
The method of analysis is the layered elastic theory, based on the JULEA program, except for non-linear analysis in the hierarchical Level 1 that uses the finite element method with the DSC2D program. The FEM is not fully calibrated and, in the short term, must be used for research only [25]. The traffic loading characterization uses load spectra of single, tandem, triple, and quad axles with multiple analysis points in the pavement structure to obtain the critical responses, as shown in Figure 11.
Figure 11
Schematic for horizontal analysis locations for regular traffic. Redrawn from [25] - not to scale
The incremental damage analysis divides the design period into sub-periods of one month or even two weeks. In each sub-period, all the layers support the predicted traffic under the predominant weather and materials conditions. The damage is accumulated to predict the progressive deterioration of the structural and functional conditions.
The MEPDG can consider a wide range of materials: Hot mix dense-graded asphalt concrete, asphalt-treated open-graded permeable base materials, cold mix asphalt, Portland cement concrete, cement-treated or lean concrete base, cement-treated open-graded permeable base materials, untreated base materials (granular base or granular subbase), lime modified or stabilized soils, subgrade soil, and bedrock.
For hot-mix-asphalt-surfaced pavements, the specific performance models are [25] and [33]:
1. Total rut depth and rutting in hot-mix asphalt, unbound aggregate base, and subgrade.
a. Plastic deformation for hot-mix asphalt (HMA) computed by Equation 18:
Where Δ
p(HMA)
is the accumulated plastic vertical deformation in the HMA layer (in.); 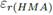 is the resilient strain calculated at the mid-depth of the HMA layer (in./in.); h
(HMA)
is the thickness of the HMA layer (in.); n is the number of axle-load repetitions; T is the pavement temperature (°F); k
z
is the depth confinement factor;k
1r
, k
2r
, and k
3r
are the global field calibration parameters (k
1r
= -3.35412, k
2r
= 0.4791, k
3r
= 1.506); and β
1r
, β
2r
, and β
3r
are local field calibration constants (1.0 by default). The depth confinement factor
is computed by Equation 19:
is the resilient strain calculated at the mid-depth of the HMA layer (in./in.); h
(HMA)
is the thickness of the HMA layer (in.); n is the number of axle-load repetitions; T is the pavement temperature (°F); k
z
is the depth confinement factor;k
1r
, k
2r
, and k
3r
are the global field calibration parameters (k
1r
= -3.35412, k
2r
= 0.4791, k
3r
= 1.506); and β
1r
, β
2r
, and β
3r
are local field calibration constants (1.0 by default). The depth confinement factor
is computed by Equation 19:
Where the coefficients C 1 and C 2 are given by Equations 20 and 21:
Where D is the depth below the surface (in.), and H HMA is the total thickness of the hot-mix asphalt (in.).
b. Plastic deformation for unbound pavement layers and the foundation or embankment soil computed by Equation 22:
Where Δ
p(soil)
is the plastic deformation of the layer (in.); n is the number of axle-load repetitions;
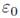 is the intercept determined from laboratory repeated load permanent deformation tests
(in./in.);
is the intercept determined from laboratory repeated load permanent deformation tests
(in./in.);
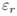 is the resilient strain imposed in the laboratory to obtain material properties
is the resilient strain imposed in the laboratory to obtain material properties
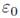 ,
,
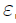 , and ρ (in./in.);
, and ρ (in./in.);
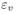 is the calculated average vertical resilient strain in the layer (in./in.); h
soil
is the thickness of the unbound layer (in.); k
s1
is a global calibration factor (k
s1
= 1.673 [granular material], k
s1
= 1.350 [fine soil]); and β
s1
is a local calibration constant (1.0 by default). The models to estimate the parameters
β,
is the calculated average vertical resilient strain in the layer (in./in.); h
soil
is the thickness of the unbound layer (in.); k
s1
is a global calibration factor (k
s1
= 1.673 [granular material], k
s1
= 1.350 [fine soil]); and β
s1
is a local calibration constant (1.0 by default). The models to estimate the parameters
β,
 , and ρ are computed with Equations 23 to 27:
, and ρ are computed with Equations 23 to 27:
Where W c is the water content (%);M r is the resilient modulus of the layer (psi); GWT is the groundwater table depth (feet); and α1 = 0.15, b 1 = 0.0, α 9 = 20.0, b 9 = 0.0. The total permanent deformation of the pavement is the summation of the contribution of all rutting-susceptible layers, as indicated in Equation 28:
Where RD total is the total permanent deformation made by the contributions of asphalt (AC), granular bases (GB), and subgrade (SG) layers. The critical responses to compute rutting are the mid-depth of each layer (or sub-layer), the top of the subgrade, and six inches below the level of the subgrade.
2. Load-related alligator cracking, or bottom-up cracking. The estimation of fatigue damage is based on Miner’s law, given by Equation 29:
Where D is the damage; T is the total number of analysis periods; n i is the actual traffic for period i; and N i is the traffic allowed under conditions prevailing in period i. The allowable number of axle-load applications needed for the incremental damage index approach to predict alligator and longitudinal fatigue cracks is given by Equation 30:
Where N
f(HMA)
is the allowable number of axle-load applications;
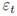 is the tensile strain at critical locations (in./in.); V
be
is the effective asphalt content by volume (%); V
a
is the air voids by volume (%); E
HMA
is the dynamic modulus of the HMA measured in compression (psi); k
f1
, k
f2
, and k
f3
are the global field calibration parameters (k
f1
= 0.007566, k
f2
= -3.9492, k
f3
= -1.281); β
f1
, β
f2
, and β
f3
are local field calibration constants (1.0 by default). The thickness correction
term (C
H
) is dependent on the cracking type and for bottom-up cracking is given by Equation 31:
is the tensile strain at critical locations (in./in.); V
be
is the effective asphalt content by volume (%); V
a
is the air voids by volume (%); E
HMA
is the dynamic modulus of the HMA measured in compression (psi); k
f1
, k
f2
, and k
f3
are the global field calibration parameters (k
f1
= 0.007566, k
f2
= -3.9492, k
f3
= -1.281); β
f1
, β
f2
, and β
f3
are local field calibration constants (1.0 by default). The thickness correction
term (C
H
) is dependent on the cracking type and for bottom-up cracking is given by Equation 31:
Where H HMA is the total thickness of the hot-mix asphalt (in). The final transfer function to calculate alligator fatigue cracking from the fatigue damage is given by Equation 32:
Where FC bottom is the bottom-up fatigue cracking (% of lane area); D bottom-up fatigue damage; C 1 = 1.0; C’1 = (-2.0 · C’2); C 2 = 1.0; C’2 = -2.40874 - 39.748 · (1 + H HMA )-2.856; and H HMA is the total thickness of the hot-mix asphalt (in.)
3. Load-related longitudinal cracking, or top-down cracking. The fatigue equation is the same as Equation 30, but the thickness correction term (C H ) is given by Equation 33:
Where H HMA is the total thickness of the hot-mix asphalt (in.). The transfer function to calculate longitudinal fatigue cracking from the fatigue damage is given by Equation 34:
Where FC bottom is the top-down fatigue cracking (feet/mile); and D top-down fatigue damage. The critical responses to compute fatigue are the surface of the pavement, 0.5 inches from the surface, and the bottom of each bound or stabilized layer.
The MEPDG also includes models to estimate non-load-related transverse cracking, fatigue cracking in chemically-stabilized bases, and reflective cracking in hot-mix asphalt overlays due to cracks and joints in existing flexible, semi-rigid, composite, and rigid pavements [33].
4. Smoothness (IRI) of pavements with unbound aggregate base and subbase. The IRI is estimated by Equation 35:
Where IRI is the IRI at any given time (m/km); IRI 0 is the initial IRI (m/km); SF is the site factor; age is the age of pavement in years; (TC L ) T is the total length of transverse cracks (m/km); COV RD is the coefficient of variation of the rut depths (assumed to be 20%); (FC) T is the fatigue cracking in wheel path (% of total lane area); (BC) T is the area with block cracking as a percent of the total lane area (user input, not modeled by MEPDG); and (LC SNWP ) M H is the length of moderate and high severity sealed longitudinal cracks outside the wheel path in meter per kilometer (user input, not modeled by MEPDG). The site factor is given by Equation 36:
Where R SD is the standard deviation of the monthly rainfall (mm); P 075 is the percent passing the 0.075 mm sieve; PI is the plasticity index of the soil (%); FI is the average annual freezing index (°C-days); P 02 is the percent passing the 0.02 mm sieve; and R m is the average annual rainfall (mm).
The MEPDG also includes models to estimate the roughness progression in pavements with asphalt-treated and chemically-stabilized bases [33].
The method comprises equations to estimate the standard deviation of the predicted distresses and their confidence intervals to compute the reliability of the structural design. However, a review of the published comparisons between the measured and estimated distress shows that the proposed models are quite dispersed, with coefficients of correlation (R²) of 0.577 for rutting, 0.275 for alligator cracking, 0.544 for longitudinal cracking, and 0.56 for IRI. Also, in the particular case of rutting, the “observed” values were proportionally distributed (not measured) between the layers from a single value of total superficial rutting [33].
In 2014, the NCHRP Synthesis 457 reported that 48 USA state highway agencies still used empirical design methods, three agencies implemented MEPDG (Indiana, Missouri, and Oregon), and 46 agencies had plans to implement the new method [34]. The implementation efforts in the USA and Canada report significant differences between the national and the local conditions, particularly about the sensitivity, bias, and standard error of the pavement distress models [35, 36]. Some local implementation programs include custom axle-load spectra, new analytical tools, and research on construction materials supported by extensive laboratory testing with good results for fatigue and roughness prediction [37, 38]. There is some concern about the quality of the distress models, particularly for the asphalt longitudinal cracking model, as reported in Minnesota, South Carolina, and Oregon [39-41].
Although the MEPDG develops the whole idea of incremental design, it lacks simplicity, and maybe some models should be reviewed for the sake of applicability for road agencies and practitioners. The future research areas for MEPDG development and improvement are robust sensitivity analysis, enhancements of the climate model and the potential impact of climate change, traffic data quality control, local calibration, and new materials [42]. The published research about MEPDG is extensive and the authors of this review do not pretend to offer a comprehensive examination of every document, but to give an overview of the mainstream lines of work.
Despite the efforts invested in the MEPDG, there is not a consensus in the USA for its implementation. For example, the California Department of Transportation (Caltrans) developed the CalME software and postulates that “its models and ideas will become part of a multistate or national long-term research and development programs” [43]. The CalME software includes the entire range of design methods used in California, from the empirical procedure and a conventional mechanistic-empirical method, based on the Asphalt Institute design criteria, to a new recursive-incremental procedure, with emphasis on the design of overlays, based on the calibration of material properties from tests with heavy vehicle simulator (HVS). The recursive-incremental procedure predicts pavement conditions in terms of fatigue cracking, reflective cracking, rutting, and smoothness in increments of time using the output from one increment as input to the next increment. CalME evaluates the reliability of pavement distress through Monte Carlo simulation [44]. A similar improvement comes from the Texas Transportation Institute (TTI) with the development of the Texas mechanistic-empirical flexible pavement design system (TxME). The TxME software follows the incremental procedure of MEPDG and CalME but differs on the materials models for cracking in asphalt mixes, considering both crack initiation and propagation for fatigue and reflective cracking, and for rutting ins asphalt mixes, granular layers and subgrade. The top-down cracking receives detailed consideration, as previously discussed in MEPDG research. As CalME, TxME improves the evaluation of the reliability of pavement distress through the Rosenblueth method, an alternative to Monte Carlo simulation [45].
Perpetual pavement design
The perpetual pavement design is a subset of mechanistic-empirical design with two main features: (1) it pursues to achieve a long life with no deep structural distress as bottom-up fatigue and rutting below the concrete layers, and (2) the method recognizes that all materials have endurance limits below which no damage will occur. Thus, the method seeks to avoid a terminal structural condition and the damage ratio (D = N expected /N allowable ) must be lesser than 1.0. The pavements are expected to perform for 50 years or more without requiring major structural rehabilitation or reconstruction [46].
The typical section of a perpetual pavement consists of three hot-mix asphalt layers over a pavement foundation: the bottom layer is a fatigue-resistant material (rich in asphalt, low air-voids, 75-100 mm thick), the intermediate layer is a rut resistant material (high modulus, 100-175 mm thick), and the wearing surface is also rut resistant, but durable and impermeable (40-75 mm thick) [47]. The tensile strain fatigue endurance limit (FEL) of asphalt mixes varies between 60 to 200 µε and is a function of the mix type [45]. The structural rutting is controlled by a vertical compressive strain in the subgrade of 200 µε or a ratio between vertical stress and unconfined compressive strength lower than 0.42.
4. Conclusions
The authors presented a five-element geotechnical-based framework for the analysis of pavements. Among the elements in that framework, the Design of pavements comprises the definition of thicknesses, qualities, and construction specifications, and the calculation of budgets considering a user-defined level of reliability.
The design of asphalt pavements for roads and streets evolved from purely empirical to mechanistic-empirical procedures through the twentieth century. The mechanistic-empirical procedures also evolved from the determination of allowable load repetitions, based on asphalt fatigue and subgrade rutting, to incremental-recursive methods that simulate the development of primary distresses, like fatigue and permanent deformation in all layers, into functional distress expressed as roughness. More remarkable yet, design reliability is evolving from the single adjustments of the traffic intensity (AASHTO 1993) or the subgrade design-value (Asphalt Institute) to simulations based on the Monte Carlo (CalME) or Rosenblueth (TxME) methods.
Table 3 summarizes the characteristics of several empirical and M-E methods, providing an overview of the development of such procedures.
The empirical method based on the CBR test is simple to use but limited by the bounds of the experimental data. Engineers should be aware of the simplifications that lead from the CBR curves in Figure 2 to extrapolations like Figure 3 based on successive transformations of the traffic categories from “typical” axle loads to traffic intensities (trucks per day) and, after the AASHO Road Test, to repetitions of the equivalent single axle load (ESAL) of 18 kips [8].
The empirical character of the AASHTO method is troublesome for the present-day design given the limitations of factors as the structural and drainage coefficients, the ESAL equations, and the limited experience with thick asphalt layers and mix design [46]. More significant, the design criteria, based on the loss of serviceability, is no longer measured nor calibrated, and its statistical relationship with modern-day International Roughness Index (IRI) is susceptible to “tweaking” as shown in Figure 4. A comparison between Figure 3 and Figure 5 shows that the difference in total pavement thickness between the NAASRA 1979 and AASHTO 1993 methods fluctuates between -53 mm to +78 mm for CBR values between 2% and 7% (assuming the known correlation Mr = 1500 * CBR) and traffic between 10 thousand and nine million of ESAL. The actual difference between the two methods is in the systematic larger thicknesses of asphalt layers, a tendency probably derived from the simplified M-E computations included in the final version of the AASHTO Guide of 1993.
The analytical or mechanistic-empirical approach gained popularity thanks to the development of the layered elastic theory and its progressive implementation in personal computers. The main advantage of mechanistic-empirical methods is the ability to adapt to changing conditions. Currently, the main breakthrough in pavement design is the shift from thickness design procedures to incremental-recursive damage analysis procedures. However, the implementation of MEPDG faces significant challenges calibrating the American national performance equations and models to local conditions. Even more, the States of California and Texas developed what could be addressed as “post-MEPDG” methodologies to overcome the drawbacks of the MEPDG in their territories, both in the theoretical framework as in the calibration and implementation processes. A subset of the M-E method is the perpetual pavement design that considers very low damage ratios to avoid deep structural distress as bottom-up fatigue cracking and rutting of the pavement foundation. All M-E methods apply, to some extent, the design principles formulated five decades ago. These principles will remain under the premise of understanding the structural behavior of pavement materials and the adaptability of the mechanistic approach.
Finally, the authors of this review believe that no design method, either the most straightforward empirical approach or the most elaborated incremental mechanistic-empirical one, is appropriate without knowledge about the fundamental design factors and calibration of the performance models for each distress mode upon consideration. The highway agencies must be encouraged to invest in research and implementation to avoid undesirable practices like employing advanced M-E software with only a few CBR tests as primary information.